The purpose of this note is to prove the following
Theorem. A Riemannian manifoldis locally conformally flat if and only if
- for
, the Weyl tensor vanishes;
- for
, the Cotton tensor vanishes.
To this purpose, let us briefly recall some definitions
The Weyl tensor. The Weyl tensor can be defined using the following formula
where 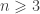 and
and 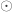 denotes the Kulkarni–Nomizu product of two symmetric (0,2) tensors. Writing the Weyl tensor in this way means that the Weyl tensor is actually a (0,4) tensor. It can be seen that the Weyl tensor can be rewritten in this form
denotes the Kulkarni–Nomizu product of two symmetric (0,2) tensors. Writing the Weyl tensor in this way means that the Weyl tensor is actually a (0,4) tensor. It can be seen that the Weyl tensor can be rewritten in this form
where the part
is called the Schouten tensor. We have the following result
Proposition. If, then
where
The Cotton tensor. The (0,3) tensor 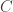 above is called the Cotton tensor. Apparently, if either the Weyl tensor or the Ricci tensor vanishes, so does the Cotton tensor.
above is called the Cotton tensor. Apparently, if either the Weyl tensor or the Ricci tensor vanishes, so does the Cotton tensor.
The Weyl and Cotton tensors under the conformal changes of metric. It is well-known that these tensors are invariant under the conformal changes of metric, that is,
under the change  for some smooth function
for some smooth function 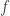 (see this note).
(see this note).
The Riemmanian curvature tensor under the conformal changes of metric. We list here the following rule
See this note for further details.
Locally conformally flat manifolds. Roughly speaking, this is to say at each point  , there exists a neighborhood
, there exists a neighborhood  of
of 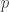 such that the conformal class of
such that the conformal class of 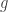 contains the flat metric in
contains the flat metric in  , that is to say
, that is to say
We are now in a position to prove the theorem.
Proof of Theorem. We first assume thatis locally conformally flat, that is,
. If
, using the formula for
we have
since the Riemmanian curvature tensor vanishes. If, we use the formula for
, we obtain
since the Ricci tensor vanishes.Conversely, if the Weyl tensor vanishes, we haveUnder the conformal change, for some, we have
Since the mappinggiven by
is injective, it suffices to show that the following equation
is locally solvable. This can be done using the following whose proof is postponed.Lemma. Provided the Weyl tensor vanishes, equationis locally solvable if and only if the following integrability condition is satis edThat is, if and only if the Cotton tensor vanishes.Recall that when; the condition follows from the Weyl tensor vanishes. This concludes the proof.

Wash your curly hair at the very least two days in a week. This article
ReplyDeletewill explain the hair product diversion conspiracy and list
ways that you can determine whether or not the beloved salon brand you saw in Walgreens last week was truly diverted merchandise or strategically placed by the powers that
be. Soon after this, use curl lotion all more than your hair, and make use of mousse and squeeze
this in your hair to that your curls seem flawless.
Also visit my web blog - hair products
Nanokeratin locks onto the hair, forming a fine,
ReplyDeletesmooth coat of keratin. As natural products don't have any side-effects, you can rest assured for healthy hair. The product should be one that is made for your type of hair, whether it be dry, oily, curly or straight.
My weblog :: hair products
37 kits (round that up to 9 total quart and a half kits).
ReplyDeleteFirst, ready mixed concrete in Sunderland can help you get your construction job done faster.
It is advantageous for the customer to compare the prices for an additional dumpster rental from the normal
waste removal company against an offer made by a collector
that will place a dumpster for a one-time pickup.
Use oils that can help keep your hair soft and manageable.
ReplyDelete- If your hair feels rough, condition it right away. You repeat the exact same procedure maybe once
or twice every week. The remaining oil in the scalp for a day and
then follow up with a shampoo the next morning.
Customers have always given Moroccan Oil products the highest ratings.
Feel free to visit my blog post - how to grow hair faster
It is indeed possible to lower high blood pressure by only taking herbal medicines and vitamins and eating certain foods that can lower high blood pressure.
ReplyDeleteIt needs to be rushed to a medical facility which is capable
of handling emergencies. It is also possible that if CLASS recipients are eventually enrolled in AHCCCS (Arizona's Medicaid program), AHCCCS will benefit financially by receiving a share of the cash payment made by the CLASS program. , one of the chiropractors in Fayetteville, is pleased to announce the implementation of programs for natural detoxification therapy. Finally, I think anyone would be inspired by Lynda's story.
Feel free to surf to my web blog ... mixobarbaric
Aside from Microsoft and Nintendo, another top competitor, Sony,
ReplyDeletewill also be releasing some top-rated games for its own console - the Playstation.
It is also a good practice to make sure that none of the climbers are more tired than the other.
However, such training programs are tough to wave through since it require tons
of learning patience and willingness to proven one’s innate skills.
The main purpose of a simulator is to understand the behavior of the
system. The instruments that lag in real
life, lag correctly, gyro drift is modeled correctly, the magnetic
compass is subject to aircraft body forces - all those things that make
real world flying a challenge are present.
Download Aplikasi Handphone Terlengkap
ReplyDeleteKlid Di sini http://an-android.blogspot.com/search/label/Games